Engineering and Science Laws Series - Kepler's Law

 

The German astronomer Johannes Kepler, who was Brahe's assistant, acquired Brahe's astronomical data and spent about 16 years trying to deduce a mathematical model for the motion of the planets. After many laborious calculations, he found that Brahe's precise data on the resolution of Mars about the Sun provided the answer. Such data are difficult to sort out because the Earth is also in motion about the Sun.
 

1.	All planets move in elliptical orbits with the Sun at one of the focal points.
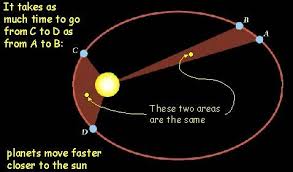
2.	The radius vector drawn from the Sun to a planet sweeps out equal areas in equal time intervals.
3.	The square of the orbital period of any planet is proportional to the cube of the semimajor axis of the elliptical orbit.

Kepler's analysis first showed that the concept of circular orbits about the Sun had to be abandoned. He eventually discovered that the orbit of Mars could be accurately described by an ellipse with the Sun at one focal point. He then generalized this analysis to include the motion of all planets. The complete analysis is summarized in three statements, known as Kepler's laws:

Half century later, Newton demonstrated that these laws are the consequence of a simple force that exists between any two masses. Newton's law of gravity, together with his development of the laws of motion, provides the basis for a full mathematical solution to the motion of planets and satellites. More important, Newton's law of gravity correctly describes the gravitational attractive force between any two masses.

Mathematical statements:

Kepler's second law

Where dA is the area swept by radius vector r in a time dt and Mp is the planet mass.

Kepler's third law

Where KS is a constant given by

MS is the Sun mass, G is universal gravitational constant and T is the time. Read more